V.2 #5 Mathematics - The Interactive Unit as a Tool in Teaching Math
Gaps in the mathematics performance of students with disabilities compared to their nondisabled peers appear in the early elementary grades and widens as students advance in grade level (Cawley, Parma, Foley, Salmon & Roy, 2001). One method to help decrease this gap is through the use of alternative representations to present material such as the progression of concrete, semi-concrete, and abstract presentations (Agaliotis, 2008, February; Bruno, 1966; Council for Exceptional Children, 1998) when teaching place value (Agaliotis, 2008, January). For students who continually struggle with the abstractness of mathematics a variation of the concrete, semi-concrete, and abstract sequence is to provide a framework in which teachers and students interact with material utilizing a variety of input and output combinations in any order. A benefit of utilizing a variety of input and output combinations is the enormous flexibility available in developing instructional interactions primarily based on students’ needs and the characteristics of the material presented.
An expanded form of alternative representation allows teachers to present information (input) by manipulating, displaying, stating, or writing new materials or concepts and students to respond (output) by manipulating, identifying, stating, or writing. This results in a combination of 16 possible interactions between the teacher and students as depicted in the Interactive Unit (IU) below (note: the cell numbers in the IU indicate the specific input/output interactions between the teacher/student respectively) (Cawley, Fitzmaurice, Goodstein, Lepore, Sedlak, & Althaus, 1974; Cawley & Reines, 1996; Cawley, Hayes, & Foley, 2008). One of the beauties of the IU is its flexibility in presenting math content and skills to students while also allowing teachers to go beyond the more traditional state and write student response formats. With this flexibility teachers can take into consideration the specific strengths and needs of students that characterize their specific disability(ies) while planning lessons. The flexibility of the IU allows teachers to differentiate lessons to meet the variety of students’ learning needs within any one class.
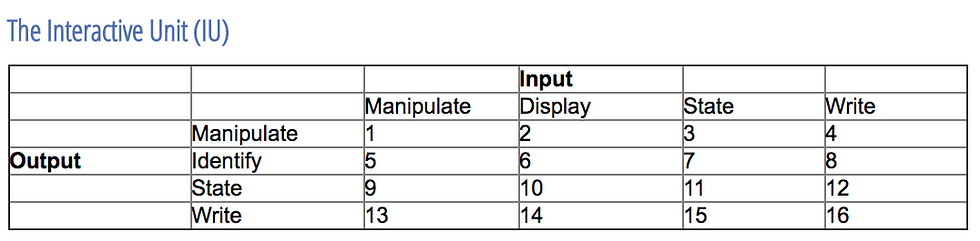
The Input dimension describes how teachers present material to students while the Output dimension indicates how students respond to the series of prompts or questions posed by the teacher. On the Input dimension, manipulate stipulates that teachers compile and then move or arrange two- or three-dimensional materials (e.g., blocks, pictures of blocks, sticks, coins, etc.) to represent a mathematical concept or skill set; display indicates that teachers show students a picture of an object (e.g., picture of a block or coin, etc.) or an object itself (e.g., a block, a coin, etc.) that represents a mathematical concept or skill set. A key distinction between manipulate and display is when the manipulative option is used the objects are moved or rearranged, (i.e., manipulated) as a way to demonstrate a concept or skill and then the objects are removed in a few seconds. If the objects remain in sight or in a fixed format then the presentation takes on the form of display, as the blocks are now in a stationary form. State and write describe the more traditional forms or methods in which mathematics is presented to students. State describes instruction in which material is presented orally by the teacher. Write specifies the teachers’ use of written symbols that consist of letters, numerals, images of squares or circles, or other mathematical symbols. The input option of write is most commonly evident in the use of worksheets or handouts in which material is presented in written form that students are asked to complete or discuss.
Students’ output options include manipulate, identify, state, and write. Manipulate requires students to move one or more items (e.g., blocks, pictures of blocks, sticks, coins, etc.) in response to a question or request posed by the teacher as a way to demonstrate their understanding of concepts. Identify requires students to point to or otherwise indicate the correct answer among a collection of possible answers. State requires students to verbalize their responses to the teachers’ question, while the write option asks students to write their responses to the teachers’ question.
The flexibility of the IU is in combining the options between the teachers’ Input and the students’ Output, which results in 16 possible types of interactions (i.e., 16 cells as indicated in the matrix). For example if an interaction is described in the form of Input/Output, then a manipulate/write interaction (i.e., cell 13) can be descriptive of an instructional interaction in which the teacher manipulates a collection of objects or pictures and the students are asked to respond by writing an equation that describes the manipulation demonstrated by the teacher. A manipulate/manipulate interaction (i.e., cell 1) could be descriptive of a situation in which a teacher demonstrates a concept such as 3 + 4 with a collection of blocks that are removed after the concept is demonstrated and the student(s) are asked to demonstrate the same concept (i.e., 3 + 4) using a different set of materials. A manipulate/identify interaction (i.e., cell 5) could be descriptive of the same presentation by the teacher while the student(s) are asked to identify (i.e., pick or mark) on a worksheet the correct math statement that corresponds with the manipulation demonstrated by the teacher. Similarly a manipulate/state interaction (i.e., cell 9) is descriptive of a situation in which the teacher engages in the same behavior, while student(s) are asked to verbalize a math statement that explains the actions of the teacher. In addition, 10 of the 16 Input/Output combinations (i.e., cells 2, 4, 5, 6, 7, 8, 13, 14, 15, and 16) can be used to create worksheets for student or classroom use. (e.g., See Foley & Cawley, 2003 and Foley, Parmar, & Cawley, 2004 for additional examples using the IU). (See Agaliotis, 2008, November— for additional suggestions on teaching division and Agaliotis, 2008, February—for suggestions on using alternative algorithms).
Students with learning disabilities often have notable difficulty generalizing what they learn to new applications or situations, yet students' ability to generalize skills is a key skill in learning mathematics. An example of one way to encourage generalization is for the teacher to present one group of students with various types of blocks; a second group of students with popsicle sticks wrapped in sets of 100's, 10's, and 1's a third group with dollar bills, dimes, and pennies; and a forth group with paper and pencil and ask each group to use the materials they are given to create a representation of 23√276
(Foley & Cawley, 2003). To further strengthen students' skills at generalization they can be asked to explain not only 'what' they did and 'why' it works mathematically.
The flexibility of the Interactive Unit allows it to be utilized with students with developmental disability, learning disabilities, or nearly any other type of disability or ability. Likewise, lessons can be tailored to students with various math abilities and skills within the same classroom and thus allows for diversification of instruction. Overall, the flexibility and usefulness of the IU as a teaching tool are nearly endless.
References
Agaliotis, I. (2008, January). Using manipulatives to enhance place value understanding and algorithmic learning in students with learning disabilities. Strategies for Successful Learning, 1(2). Retrieved January 9, 2009, from http://www.ldworldwide.org/members/professionals/ssl/SSL01-08_agaliotis.cfm.
Agaliotis, I. (2008, February). Alternative algorithms or struggling learners. Strategies for Successful Learning, 1(3). Retrieved January 9, 2009, http://www.ldworldwide.org/ldinformation/educators/ssl/SSL02-08_agaliotis.html.
Agaliotis, I. (2008, November). Teaching division to students with learning disabilities. Strategies for Successful Learning, 2(2). Retrieved January 9, 2009, from http://www.ldworldwide.org/ldinformation/educators/ssl/SSL11-08_agaliotis.html.
Bruner, J. (1966). Toward a theory of instruction. Cambridge, MA: Harvard University Press.
Cawley, J., Fitzmaurice, A. M., Goodstein, H., Lepore, A., Sedlak, R., & Althaus, V. (1974). Project MATH. Tulsa, OK: Educational Progress Corporation.
Cawley, J. F., Hayes, A., & Foley, T. E. (2008). Teaching math to students with learning disabilities: Implications and solutions. Lanham, MD: Rowman & Littlefield Education in Partnership with Learning Disabilities Worldwide.
Cawley, J., Parmar, R., Foley, T. E., Salmon, S., & Roy, S. (2001). Arithmetic performance of students with mild disabilities and general education students on selected arithmetic tasks: Implications for standards and programming. Exceptional Children, 67(3), 311–328.
Cawley, J. F., & Reines, R. (1996). Mathematics as communication: Using the interactive unit. Teaching Exceptional Children, 28(2), 29-34.
Council for Exceptional Children. (1998). What every special educator should know (3rd ed.). Reston, VA: Author.
Foley, T. E., & Cawley, J. F. (2003). About the mathematics of division: Implications for students with disabilities. Exceptionality: A Special Education Journal, 11(3), 131–149.
Foley, T. E., & Parmar, R. S., Cawley, J. F. (2004). Expanding the agenda in mathematics problem solving for students with mild disabilities: Alternative representations. Learning Disabilities: A Multidisciplinary Journal, 13(1), 7–16.
